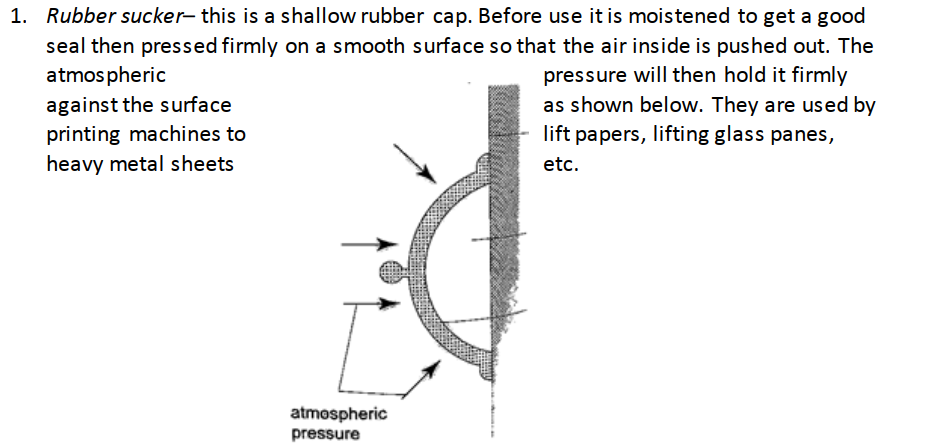
PHYSICS HIGH SCHOOL NOTES FOR KCSE PREPARATIONS
Specific ObjectivesBy the end of this topic, the learner should be able to:
CELLS AND SIMPLE CIRCUITS (12 LESSONS)
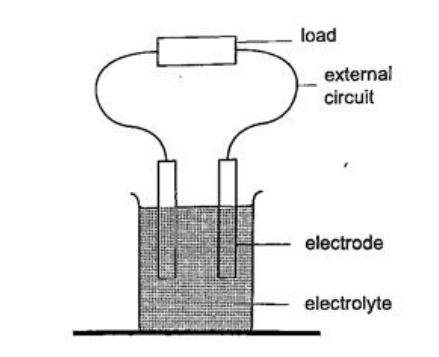
CELLS AND SIMPLE CIRCUITS.IntroductionWork done per unit charge is called electrical potential. Current is the flow of charge. For current to be continuous, potential difference between the two points must be sustained. Sources of continuous currents.In this process work is continuously done in moving electrons against a repulsive force. A device in which the potential difference is sustained is called a cell. A cell is a source of continuous current.The end of a cell with a higher potential (fewer electrons)is called the positive terminal while the end with lower potential (higher electrons) is called the negative terminal. 1. Chemical sourcesA good example is the electrochemical cell where simultaneous oxidation-reduction process occurs between the electrolyte and the electrodes.An external circuit is used to transfer the electrons. Examples of electrochemical cells are the primary cells i.e. the dry cell and Daniel cell. The reactants must be replaced after supplying a given amount of energy. The second type is the secondary cell or storage cell where the chemical reaction is reversible i.e. the lead-acid battery and nickel-cadmium cell. The third type is the fuel cell where chemical energy supplied is continuously converted into electrical energy i.e. hydrogen-oxygen cell used in spacecraft.
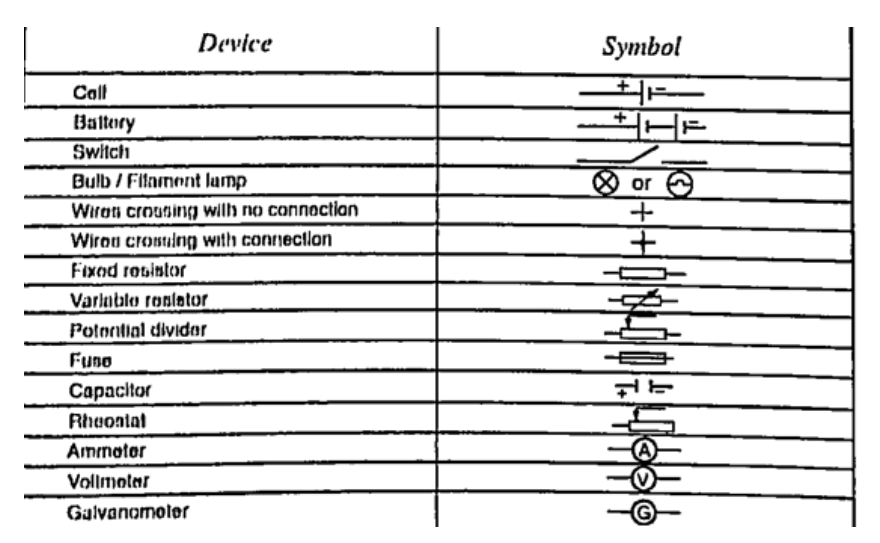
2. Thermoelectric sourcesA good example is the thermocouple where p.d is sustained by the continuous heating which keeps the terminals at different temperatures.3. Solar sourcesThis occurs when some semi-conductor material called P and N type absorbs light at their transition region and gain energy enough to move electrons just like in cells. They are used in spaceships, calculators, lighting, etc.DC circuitsConventionally current is a flow of positive charge and flows from the positive terminal to the negative terminal. A dc current is the flow of current in one direction that is from the positive terminal to the negative terminal when the loop is closed.Circuit symbolsThe following symbols are used in electrical circuits.
Potential difference and currentPd is the work done by moving an electron from one point of a conductor to another. Current is by definition the rate of flow of charge. Current = charge / time The SI unit for current is the ampere, A.
1 A = 1 Coul/sec 1 milliampere (mA) = 10-3 A 1 microampere (µA) = 10-6 A Examples 1. The current in a single loop is 3.0 A. How long would it take for a charge of 3600 coulombs to flow? Solution
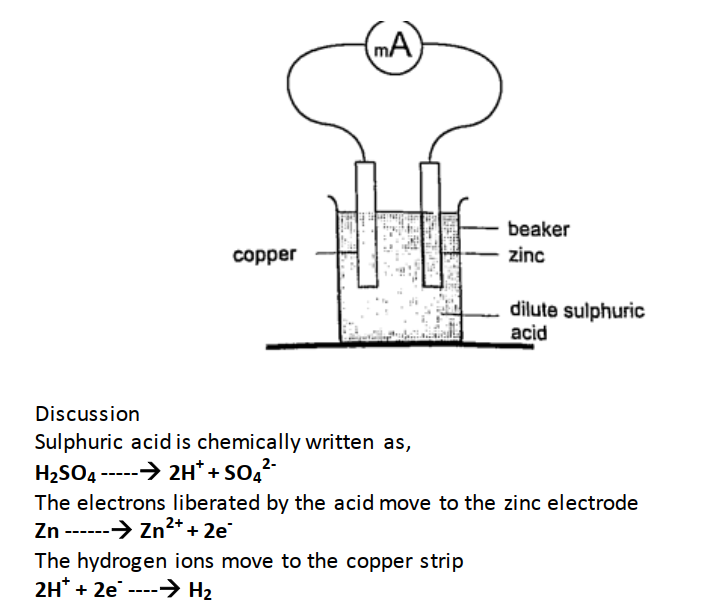
Current = charge / time Time = charge / current => 3600 / 3 = 1200 seconds = 20 minutes. Primary cells This is a cell formed by dipping two different metals into an electrolyte. Experiment: making a simple cell Procedure
Copper strip therefore becomes positively charged while the zinc becomes negatively charged electrode.
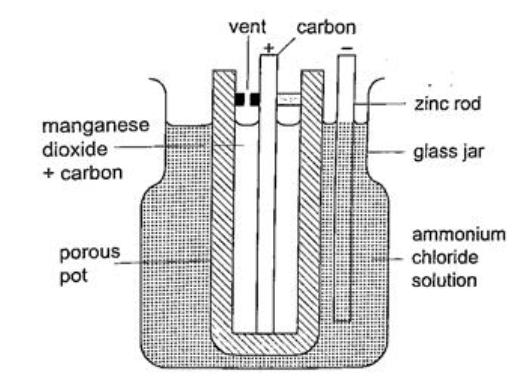
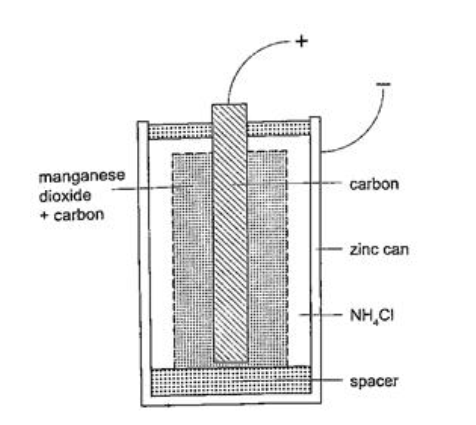
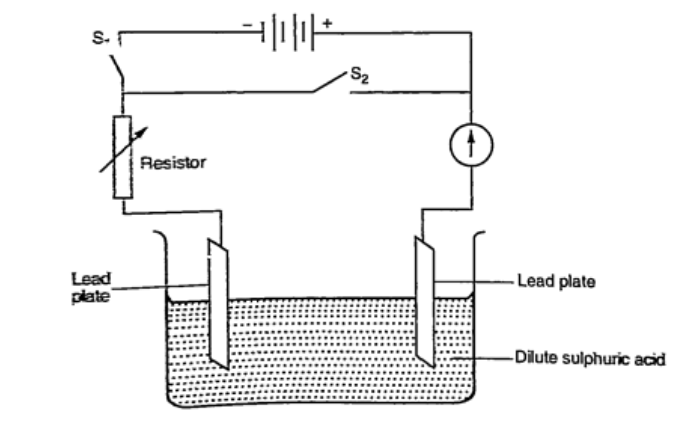
The accumulation of bubbles around the copper strip is called polarization. The bubbles formed around the zinc strip is the reaction of acid with zinc impurities and is called local action. Polarization produces insulation between the strip and the acid cutting off production of current eventually. This is known as the internal resistance of the cell. Local action eats away the zinc strip and a mercury coat is applied to prevent this (amalgamation). Polarization and local action are the main defects of simple cells. The Leclanche’ cellIn this cell carbon rod is used as the positive terminal and zinc as the negative electrode.The electrolyte is ammonium chloride solution (NH4Cl). No polarization since it is reduced by use of manganese (IV) oxide (MnO2) which oxidizes hydrogen into water. Local action still occurs. They are used in operating bells and telephone boxes. The dry cellIt is referred to as dry because it contains no liquid. The ammonium solution is replaced with ammonium chloride jelly or paste, the manganese (IV) oxide and carbon powder are used as the depolarizer. The hydrogen gas produced is oxidized to water which eventually makes the cell wet after use. They are used in torches, radios calculators etc.Secondary cellsThey are also called storage cells since they store electrical charge as chemical energy.Experiment: To charge and discharge a simple secondary cell Procedure
DiscussionWhen charging oxygen is produced at the anode and hydrogen at the cathode. The oxygen reacts with lead to form lead (IV) oxide which is deposited at the anode. The hydrogen formed has no effect.When discharging current flows in opposite direction with oxygen being formed at the cathode and hydrogen at the anode. The colour of the positive electrode changes from brown to grey. Lead-acid accumulator.A 12V accumulator has six cells connected in series. Each cell has several plates forming lattice grid with positive plates carrying lead (IV) oxide and the negative plates having spongy lead. They are placed close to one another with an insulating sheet separating them.They are rated in ampere-hours i.e. 30 Ah means that it can supply 1 ampere for 30 hours or 2 amperes for 15 hours etc.
Example
A battery is rated at 30 Ah. For how long will it work if it steadily supplies a current of 3 A? Solution Q = I t, hence t = Q / I => 30 / 3 = 10 hours. Alkaline accumulatorsPotassium hydroxide (KOH).Nickel hydroxide (Ni (OH)) forms the positive electrode while iron forms the negative electrode.They are two types nickel cadmium (NiCd) and nickel iron (NiFe). They are used in ships, hospitals and buildings where large currents are required for emergencies. Advantages of alkaline accumulators over lead-acid accumulators
Disadvantages
0 Comments
RECTILINEAR PROPAGATION AND REFLECTION AT PLANE SURFACES.IntroductionObjects that produce their own light are known as luminous objects i.e. the sun, torch lamps etc. objects that do not produce their own light are called non-luminous objects i.e. the moon. Opaque objects are those which do not allow light to pass through them.Translucent materials are those which allow light to pass through them but we cannot see through them i.e. church glass and bathroom glass. Transparent materials are those which allow light to pass through them and we can see through them i.e. window panes, car windows etc. A ray is the direction of the path followed by light. A beam is a group of rays travelling together.
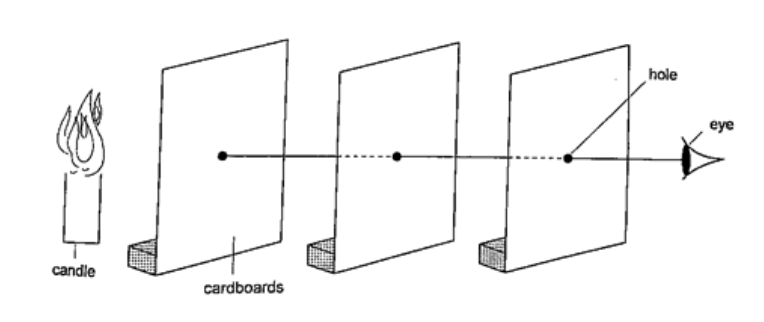
Experiment: light travels in straight lines
Procedure
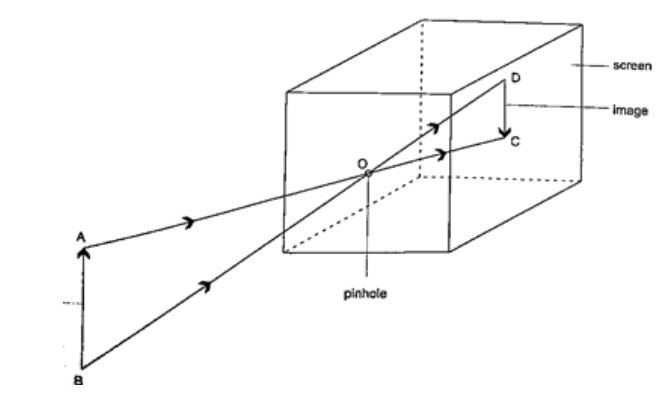
DiscussionWhen one cardboard is displaced or moved slightly the flame cannot be seen at the other end. This shows that light travels in a straight line. This principle is applied in the following,Pinhole cameraIt consists of a closed box with a small hole on one face and a screen of tracing paper/ frosted glass on the opposite face as shown. An image will be formed on the screen. Since light travels from one point of the object through the hole an image will be formed on the opposite screen of the box.If the object is near the hole it is magnified while diminished if away from the hole. Magnification is therefore the ration of the image to object height, expressed as, Magnification
= height of image/ height of object or
= distance of image from pinhole/ distance of object from pinhole
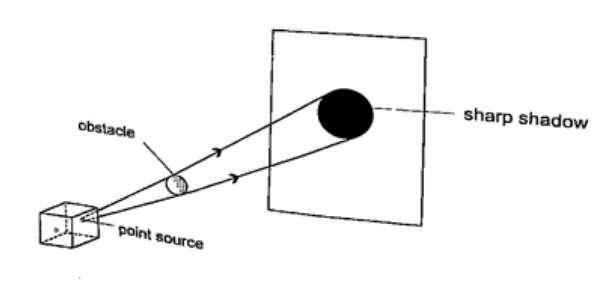
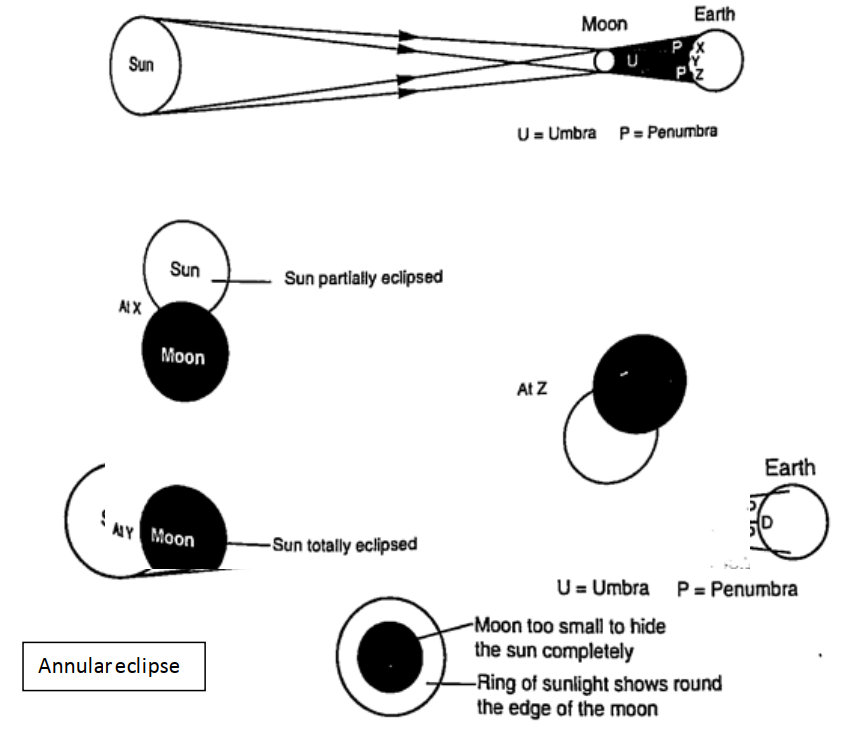
ShadowsShadows are formed when an opaque object is placed between a source of light and a screen. When the shadow is big a dark patch at the centre is formed (umbra) while a surrounding lighter patch called penumbra is formed.EclipsesEclipse of the sun (solar eclipse)This occurs when the moon is between the earth and the earth. The shadow of the moon falls on the earth’s surface. Sometimes the distance is large for the shadow to reach the earth and when this happens an annular eclipse occurs.
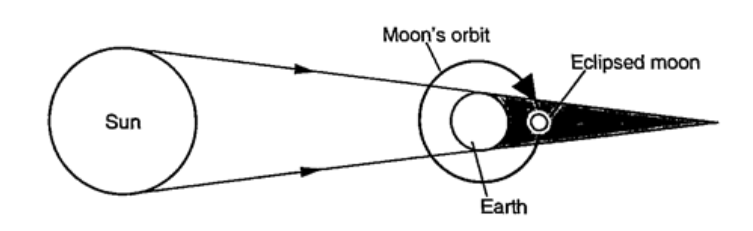
Eclipse of the moonIt is also known as lunar eclipse and occurs when the earth is between the sun and the moon. The shadow of the earth falls on the moon.Examples1. Calculate the height of a building 300 m away from a pinhole camera which produces an image 2.5 cm high if the distance between the pinhole and the screen is 5.0 cm.Solution
Object distance = 300 m, image height = 2.5 cm, image distance = 5.0 cm. Object height/ image height = object distance/ image distance Object height = (30,000 × 2.5) / 5.0 = 15,000 cm = 150 m. 2. The length of a pinhole camera is 25.0 cm. An object 2.0 cm is placed 10.0 m from the pinhole. Calculate the height of the image produced and its magnification. Solution
Image height = (image distance × object height) / object distance
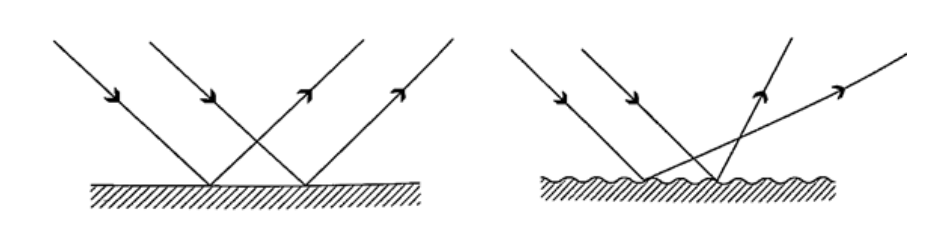
= (25 ×200) / 10 = 500 cm or 5 m. Magnification = image distance / object distance = 25 /10 = 2.5 Reflection from plane surfacesDiffuse and regular reflectionRegular reflection occurs when a parallel beam of light falls on a plane mirror band reflected as a parallel beam. They occur on polished surfaces. A diffuse reflection occurs on rough surfaces where a parallel beam of light is reflected in all directions.THE THREE LAWS OF REFLECTIONAny mirror obeys the three laws of reflection, flat, curved, convex or concave.The three laws of reflection are
Images formed by reflection from plane surfacesCharacteristics of images formed in a plane mirror
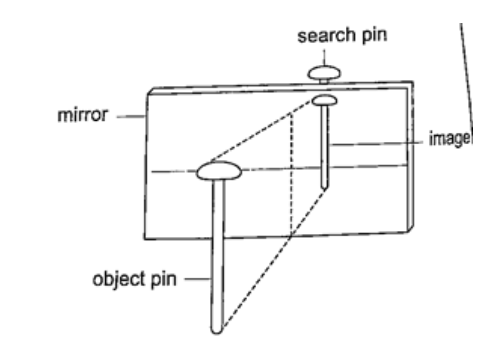
Location of an image by the non-parallax methodParallax is the apparent relative motion of two objects due to the movement of the observer. It only occurs when the objects are at a distance from one another. This can be used to find the position of images in plane mirrors.Experiment: To find the position of an image of a pin by non-parallax method Procedure
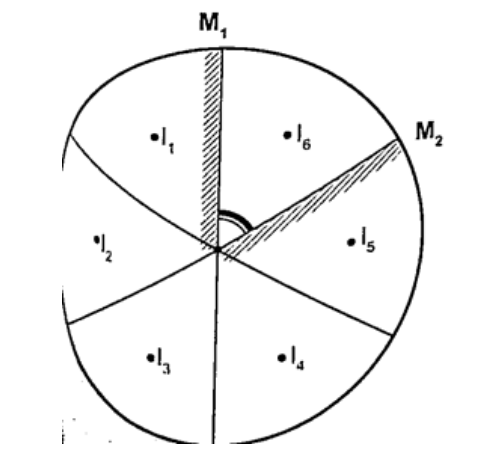
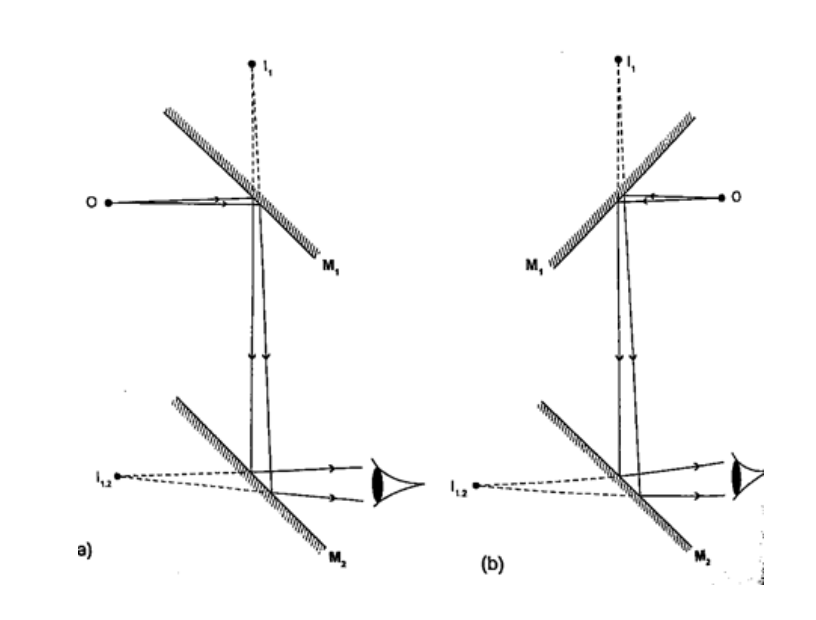
Mirrors at an angleWhen mirrors are placed at an angle several images are obtained depending on the angle between them. If the angle is 600 the images formed will be five. We use the following formula to find the number of images
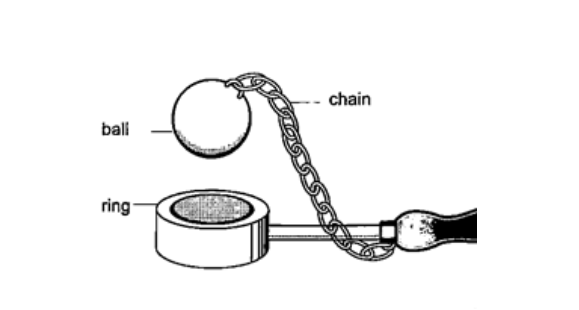
n = (3600 / θ) – 1 When mirrors are parallel then the images formed are infinite. KaleidoscopeIt applies the principle of mirrors at an angle. Consists of two mirrors arranged at an angle of 600 to one another inside a tube. The bottom has a ground-glass plate with brightly coloured glass for allowing light. When one observes through the tube five images are seen.The periscopeThis consists of two mirrors arranged at an angle of 450 as shown. This principle is used in periscopes (prisms) and telescopes.THERMAL EXPANSION.IntroductionTemperature is the degree of hotness or coldness of a body. Both Celsius scale (0C) and Kelvin scale (thermodynamic scale) are used to measure temperature. The Kelvin scale is also known as the absolute scale temperature and is measured from absolute zero (0 K).Expansion of solidsWhen solids are heated they expand. The expansion is so small such that we can’t see them.The following experiments will demonstrate actual expansion of solids. Experiment 1:- Ball and ring experiment Procedure
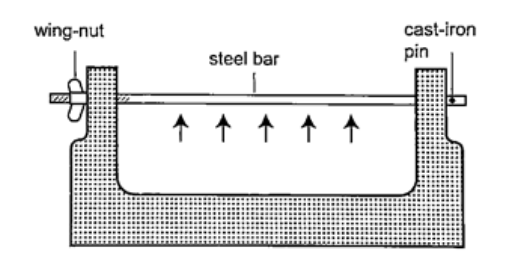
DiscussionWhen the ball is heated it expands and increases in diameter. This makes the ball not to pass through the ring. After cooling it is found that the ball slips through the ring easily again.Experiment 2:- The bar-breaker Procedure
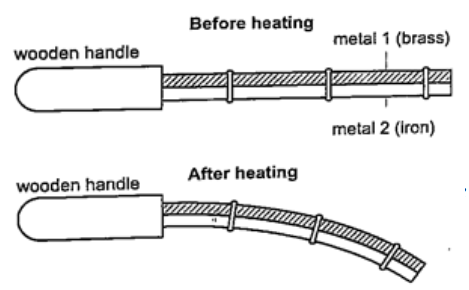
DiscussionWhen the bar cools the cast-iron pin breaks. This shows that as the bar cools it contracts and strong forces pull against the pin. These forces makes the pin to break. Experiment 3:- Heating a bimetallic strip Procedure
DiscussionWhen a brass-iron bimetallic strip is heated it bends towards the iron. This means that brass expands more than iron and this causes the strip to bend towards the iron side. This shows that different materials expand at different rates when heated.Applications of the expansion of solids
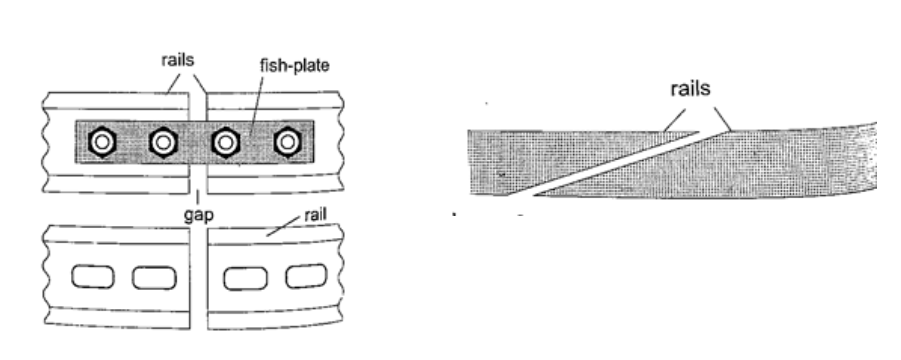
1. Construction of railway lines– an expansion joint is allowed between any two rails to accommodate expansion. A fish plate is used to join two rails. Modern railway system use the overlapping joint at the end of rails.
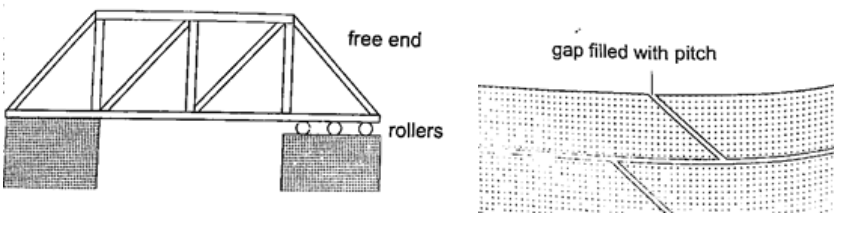
2. Construction of bridges and roof tops (steel girders) – for bridges one side has rollers while the other is fixed to allow for expansion. Concrete slabs are also laid on the ground leaving space filled with pitch to allow for expansion.
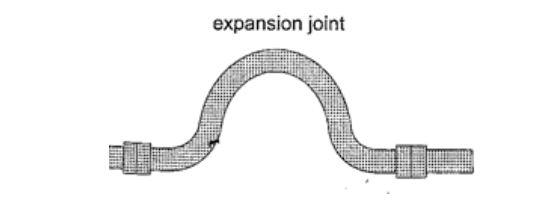
3. Hot water pipes – pipes carrying hot water (steam) from boilers are fitted with expansion joints for expansion.
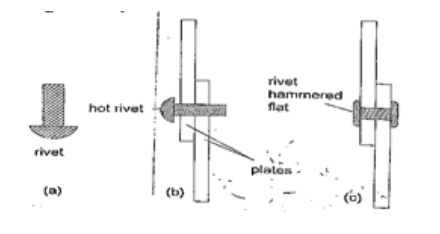
4. Riveting – used to join two pieces of metal together i.e. bimetallic strips, car bodies, drums etc. Fitting rail cart wheel using heat uses the principle of rivets. Bimetallic strips are used in thermostats (control temperature) – electric iron box, alarm systems, car flasher units etc.
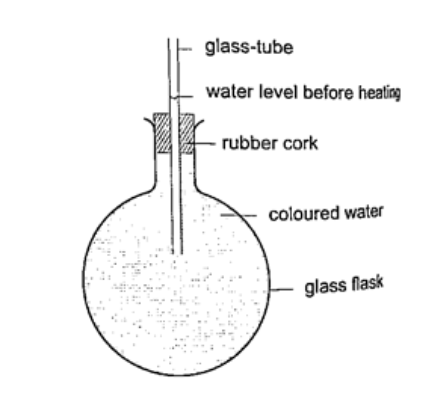
Expansion of liquids and gases.Expansion of liquids.Liquids expand more than solids so it is easy to observe and see clearly as they expand. We use the hot water bottle to demonstrate the expansion of water. Water is put in the bottle as shown below.
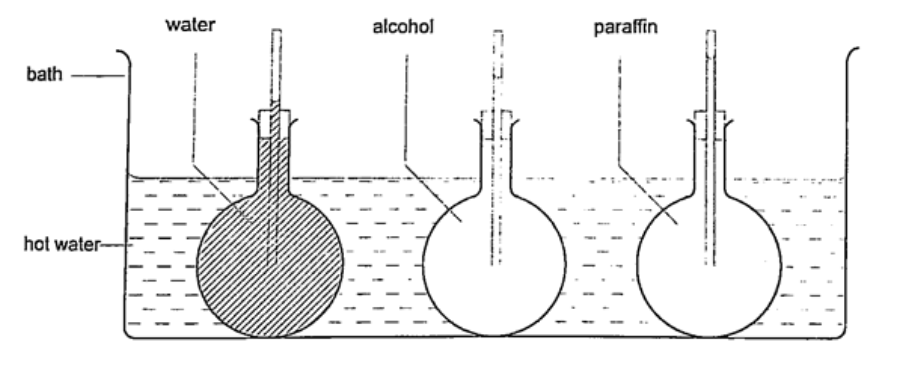
When the bottle is immersed in hot water, initially there is a drop in the level of water in the glass tube then it steadily rises after a while. This shows that liquids expand with increment in volume as shown by the hot water bottle. Different liquids expand at different rates as shown below.
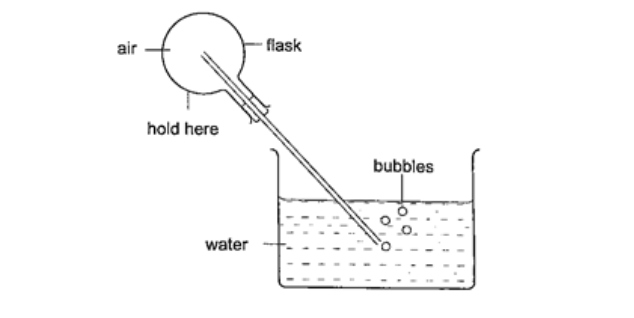
Expansion of gasesThey are the easiest to observe since they expand the most.Experiment: - Expansion of air Procedure
DiscussionThe heat produced by the hands makes the air inside the flask to expand. This makes the volume to increase and therefore force the excess air out as bubbles.Applications of the expansion of gases and liquids.
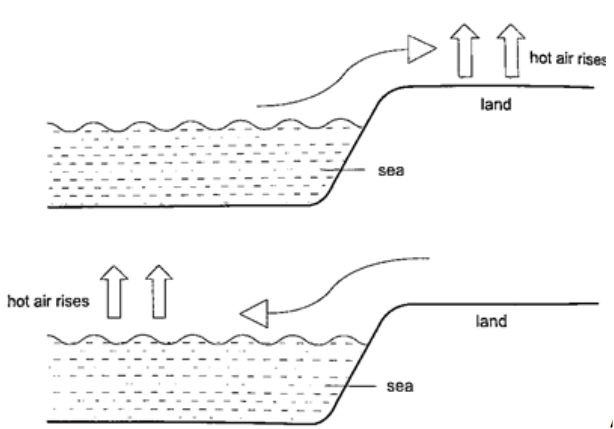
1. Land and sea breeze– during the day the land is heated by the sun causing the air above it to expand. The air becomes less dense therefore it rises. The space left is quickly filled by another cool air (generally from the sea since the land gets hot faster). This causes a cool breeze form the sea during the day. At night the land loses heat faster than the sea. The air above the sea rises since it is less dense and cool air from the land rushes to fill the gap. This causes a breeze blowing from the land to the sea.
Thermometers
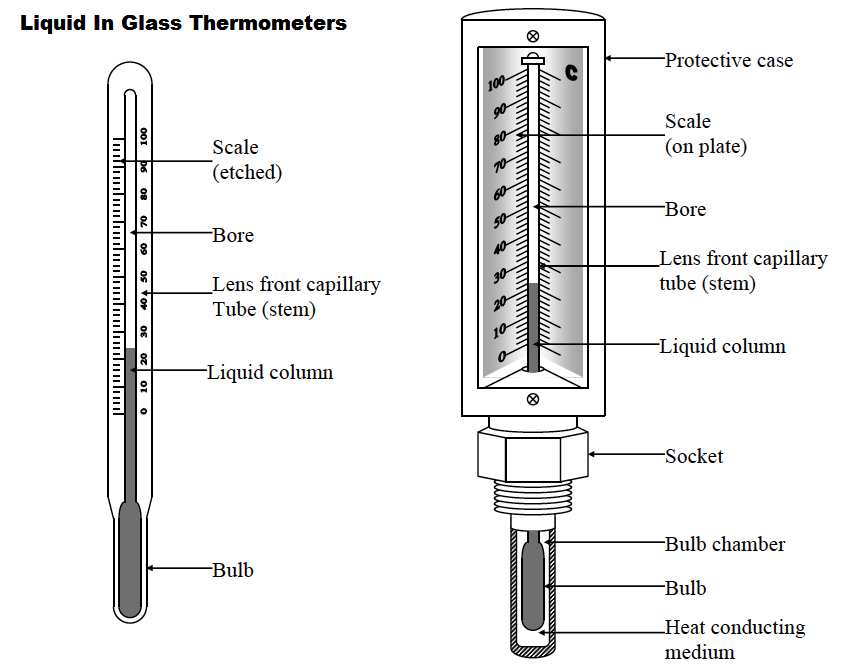
1. Liquid-in-glass thermometer–this applies to the expansion of a liquid in a thin-walled glass-tube. The liquid moves up the tube when the bulb is heated. The liquid must be a good conductor, visible and be able to contract and expand quickly and uniformly over a wide range of temperatures. It should also not stick on the sides of the tube.
Liquids commonly used are mercury and coloured alcohol. The scale is obtained by choosing two temperature points called fixed points. In Celsius lower point is taken to be 0oC (when placed in ice) and the upper point as 100oC (boiling steam). The two points are therefore divided into 100 equal parts (calibration). The melting and boiling points of both mercury and alcohol are (-39oC – 357 oC) and (-112 oC - 78 oC) respectively.
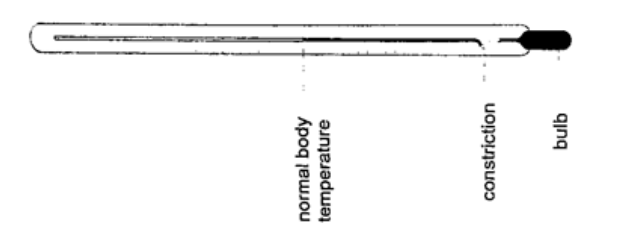
2. Clinical thermometer– this is a special type of mercury-in-glass thermometer used to measure body temperature. Since body temperature is normally 37 oC the scale is only a few degrees below and above 37oC. It has a constriction which prevents mercury from going back after expansion for convenient reading of temperature.
This thermometer has a narrow bore for greater sensitivity and accuracy.
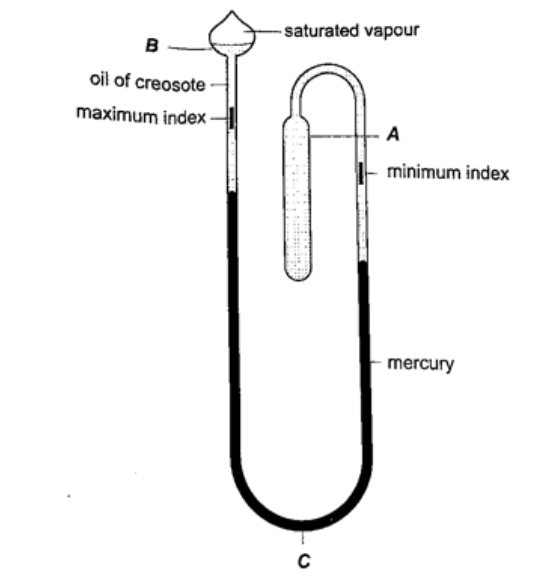
3. Six’s maximum and minimum thermometer– it is used to measure temperature of surroundings of an area or a place. It can record both maximum and minimum temperatures attained.
Consists of a large bulb (A) containing oil of creosote connected to U-shaped stem which connects to a second bulb (B) containing the same liquid. The base (C) contains a thin thread of mercury. The range of this thermometer is between -20 oC and 50 oC. After each reading the indices are pulled down to the level of mercury by use of a magnet.
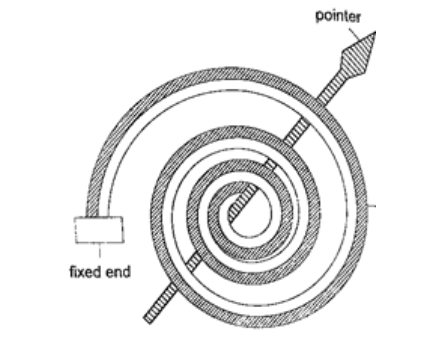
4. Bimetallic thermometer– it is made up of a bimetallic strip with one end fixed and the other connected to a pointer. Metals used are usually brass and invar. As temperatures increase the strip unwinds and moves the pointer over a calibrated scale. It is used to measure high temperatures.
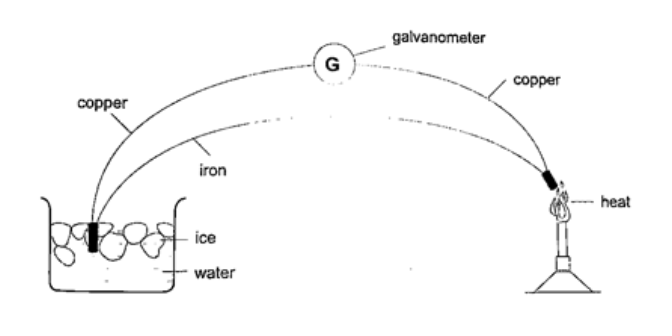
5. Thermocouple thermometer– thermocouple is a junction made of copper and iron looped at both ends. In practice a sensitive millivoltmeter is used instead of a galvanometer. A cold junction is maintained in melting ice (0oC) while the other junction is heated steadily. This thermometer does not apply the principle of expansion.
Unusual expansion of water.
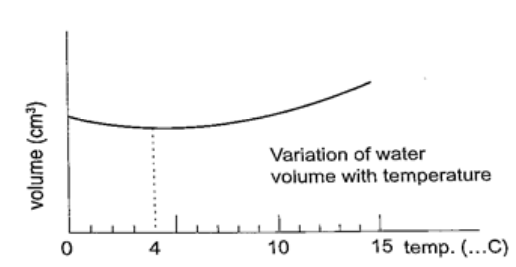
If water is heated let’s say from -15oC it expands normally like any solid but only up to 0oC.
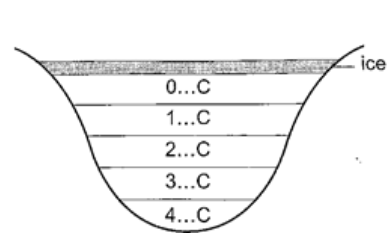
At this point it starts to melt and it contracts. This contraction will be observed up to 4oC. When heated further water starts to expand up to boiling point. This is the unusual expansion of water. This makes the top of water to freeze (0oC) in temperate countries allowing the one below to remain liquid (4oC). This supports marine life during winter. Molecules and heat.1. Solids – when heated molecules in solids absorb heat energy and vibrate. They push against one another and this causes expansion. Further expansion may result to collapse as melting in ice. 2. Liquids – besides vibrating particles in a liquid move short distances. As they move they collide by hitting each other and this results to more expansion. For boiling to occur molecules absorb enough energy to be able to escape from the liquid. 3. Gases – individual particles are free of one another and in rapid motion. When heated there are collisions with the walls of the container. This results to high pressure in the container. PRESSUREPressure is defined as the force acting normally (perpendicularly) per unit area. The SI units for pressure is newton per metre squared (N/m2). One Nm-2 is known as one Pascal (Pa). Pressure = normal force / area or pressure = thrust / area. Another unit for measuring pressure is the bar. 1 bar = 105 N/m2. 1millibar = 100 N/m2. Calculating pressureExamples 1. A rectangular brick of weight 10N, measures 50 cm × 30 cm × 10 cm. calculate the values of the maximum and minimum pressures which the block exert when resting on a horizontal table.
Solution Area of the smallest face = 0.3 × 0.1 = 0.03 m2. Area of the largest face = 0.5 × 0.3 = 0.15 m2. Maximum pressure = 10 N / 0.03 = 3.3 × 102 N/m2. Minimum pressure = 10 N / 0.15 = 67 N/m2. 2. A man of mass 84 kg stands upright on a floor. If the area of contact of his shoes and the floor is 420 cm2, determine the average pressure he exerts on the floor. (Take g = 10 N/Kg)
Solution Pressure = force / area = 840 / 0.042 = 20,000 Nm-2. Pressure in liquids.The following formula is used to determine pressure in liquids.Pressure = h ρ g, where h – height of the liquid, ρ – density and g – is force of gravity. Examples 1. A diver is 10 m below the surface of water in a dam. If the density of water is 1,000 kgm-3, determine the pressure due to the water on the diver. (Take g = 10 Nkg-1)
Solution Pressure = h ρ g = 10 × 1000 × 10 = 100,000 Nm-2. 2. The density of mercury is 13,600 kgm-3. Determine the liquid pressure at a point 76 cm below the surface of mercury. (Take g = 10 Nkg-1)
Solution Pressure = h ρ g = 0.76 × 13,600 × 10 = 103,360 Nm-2. 3. The height of the mercury column in a barometer is found to be 67.0 cm at a certain place. What would be the height of a water barometer at the same place? (Densities of mercury and water are 1.36 × 104 kg/m3and 1.0 × 103 kg/m3respectively.)
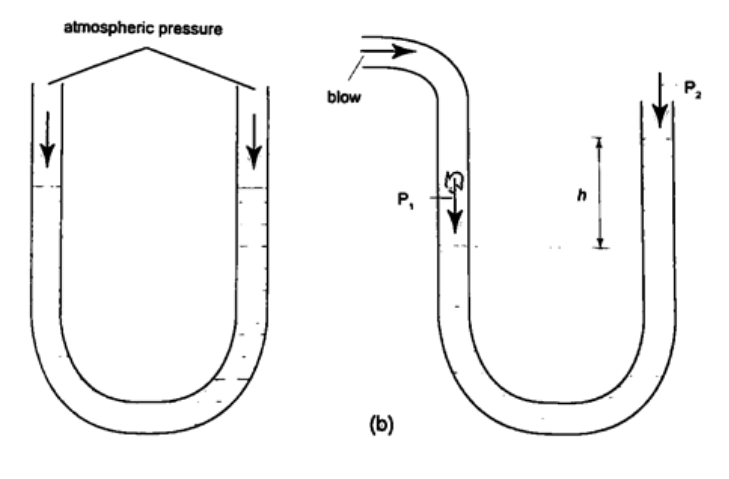
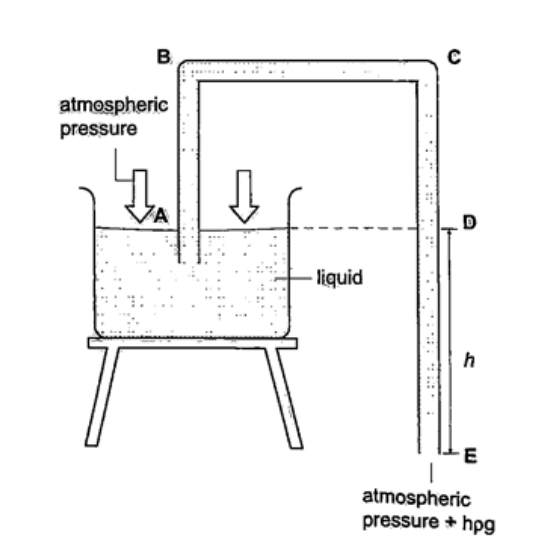
Solution Let the pressure due to water be h1ρ1g1 = h ρ g, hence; h1 = h ρ / ρ1= (6.7 × 10-1) × (1.36 × 104) = 911.2 cm or 9.11 m. U-tube manometerIt is a transparent tube bent into U-shape. When a liquid is poured into a u-tube it settles at equal level since pressure depends on height and they share the same bottom. Consider the following diagrams;
For the levels to differ the pressure P1 must be greater than P2, hence P1 =P2 + hρg.
If P1 is the lung pressure, P0 is the atmospheric pressure, then if the difference is‘h’ then lung pressure can calculated as follows. P1 =P0 + hρg. Example A man blows into one end of a U-tube containing water until the levels differ by 40.0 cm. if the atmospheric pressure is 1.01 × 105 N/m2 and the density of water is 1000 kg/m3, calculate his lung pressure. Solution Lung pressure = atmospheric Pressure + liquid pressure P1 = P0 + hρg. Hence P1 = (1.01 × 105) + (0.4 × 10 × 1000) = 1.05 × 105 N/m2. Measuring pressure
1. Simple mercury barometer– it is constructed using a thick walled glass tube of length 1 m and is closed at one end. Mercury is added into the tube then inverted and dipped into a dish containing more mercury. The space above the mercury column is called torricellian vacuum. The height ‘h’ (if it is at sea level) would be found to be 760 mm. Atmospheric pressure can be calculated as, P = ρ g h => where ρ (mercury)- 1.36 × 104 kg/m3, g- 9.81 N/kg, h- 0.76 m. Then P = (1.36 × 104) × 9.81 × 0.76 = 1.014 × 105 Pa.
NOTE- this is the standard atmospheric pressure, sometimes called one atmosphere. It is approximately one bar.
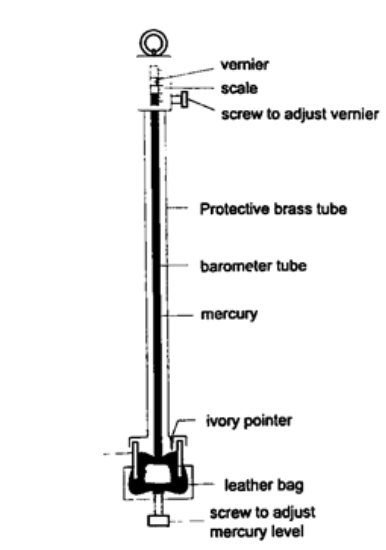
2. Fortin barometer – this is a more accurate mercury barometer. The adjusting screw is adjusted first to touch the mercury level in the leather bag.
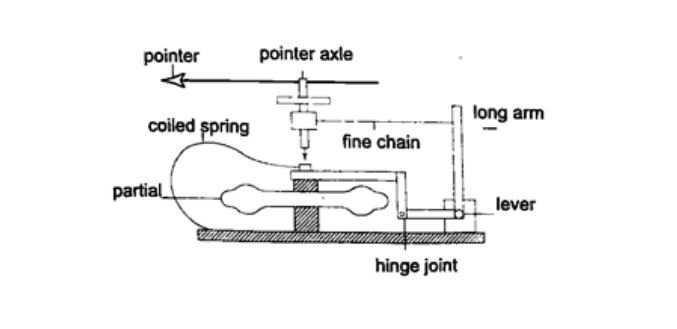
3. Aneroid barometer – increase in pressure causes the box to contract, the movements are magnified by the system of levers and is transmitted to the pointer by the fine chain and this causes the pointer to move. The scale is suitably calibrated to read pressure. Since pressure falls or rises as altitude falls or rises, the pointer can also be calibrated to read altitude.
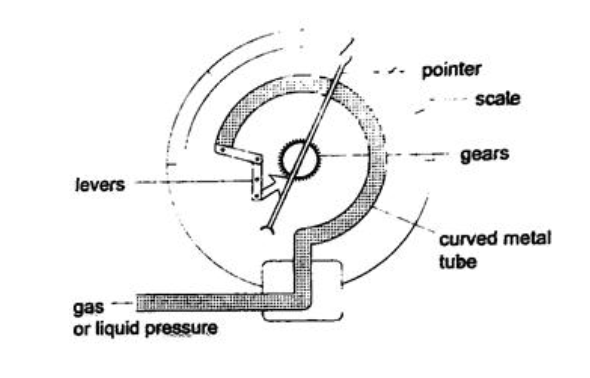
4. Bourdon gauge – it is also called gauge pressure and is used in gas cylinders. When air is blown into the rubber tube, the curved metal tube tries to straighten out and this causes movement which is transmitted by levers and gears attached to a pointer. This gauge can measure both gas and liquid pressure.
Examples
1. The height of the mercury column in a barometer is found to be 67.0 cm at a certain place. What would be the height of a water barometer at the same place? (densities of mercury- 1.36 × 104 kg/m3 and water- 1.0 × 103 kg/m3). Solution Let the pressure due to water be h1 ρ1 g1 and that of water be h ρ g. Then h1 ρ1 g1 = h ρ g. Hence h1 = (6.7 × 10-1) × (1.36 × 104) / 1.0 × 103 = 911.2 cm or 9.11 m.
Application of pressure in gases and liquids.
2. Drinking straw– when a liquid is drawn using a straw air is sucked through the straw to the lungs. This leaves the space in the straw partially evacuated. The atmospheric pressure pushing down the liquid in the container becomes greater than the pressure inside the straw and this forces the liquid into your mouth.
3. The syringe– they work in the principle as the straw. They are used by the doctors in hospitals for giving injections.
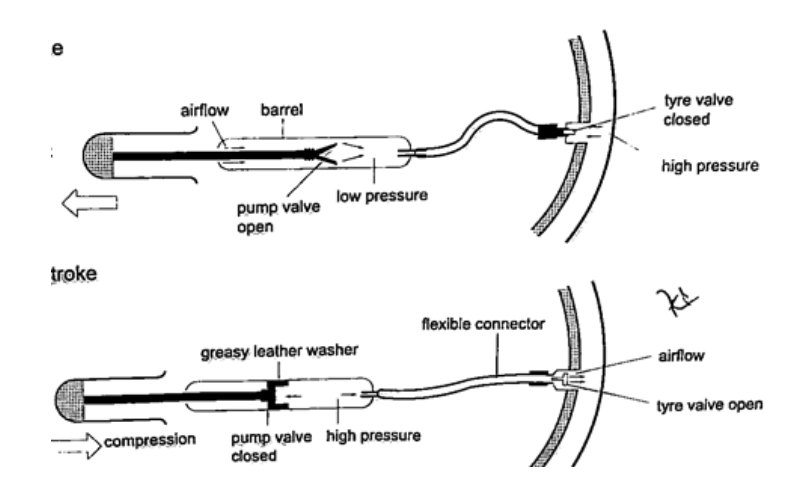
4. Bicycle pump– it uses two valves, one in the pump (greasy leather) and the other in the tire. When the handle is pushed in, the pressure inside the barrel becomes greater than the one in the tire and this pushes air inside. The valve in the tire is made such that air is locked inside once pumped.
5. The siphon– it is used to empty tanks which may not be easy to empty by pouring their contents out. The tubing must be lowered below the base of the tank. The liquid flows out due to pressure difference caused by the difference in height (h ρ g).
6. Lift pump.
7. Force pump. Transmission of pressure in liquids and gases.It was first recognized by a French mathematician and physicist called Blaise Pascal in the 17th century. Pressure is equally distributed in a fluid and equally transmitted as shown in the following, a) Hydraulic brake system – the master cylinder transmits pressure to the four slave cylinders on each wheel. The cylinders contain brake fluid. Fluid is used because liquids are almost incompressible. When force is applied in the pedal the resulting pressure in the master cylinder is transmitted to the slave cylinders. This forces the piston to open the brake shoes which then pushes the brake lining against the drum. This force the rotation of the wheel to slow down. It is important to note that pressure is equally distributed in all wheels so that the car doesn’t pull or veer to one side.
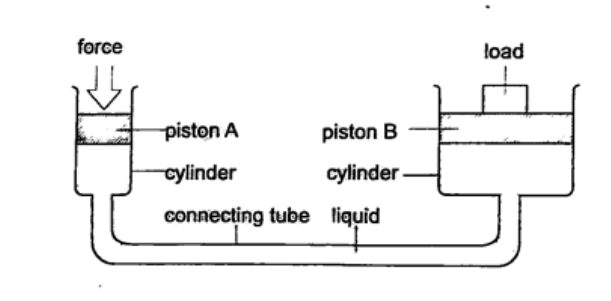
b) Hydraulic press – it consists of two pistons with different cross-sectional areas. Since pressure is transmitted equally in fluids, when force is applied in one piston it is transmitted to the other piston. The smaller piston is called the force while the bigger piston is called the load. They are used to lift heavy loads in industries, bending metals and sheets etc.
Examples
1. The area of the smaller piston of a hydraulic press is 0.01 m2 and that of the bigger piston is 0.5 m2. If the force applied to the smaller piston is 2 N, what force is transmitted to the larger piston? Solution Pressure = force / area – hence P = 2 / 0.01 = 200 Pa. Force = Pressure × Area = 200 × 0.5 = 100 N. 2. The master cylinder piston in a car braking system has a diameter of 2.0 cm. The effective area of the brake pads on each of the four wheels is 30 cm2. The driver exerts a force of 500 n on the brake pedal. Calculate: a) The pressure in the master cylinder b) The total braking force in the car. Solution a) Area of the master cylinder – π r2 = 3.14 cm2 Pressure = force /area = 500 / 3.14 × 10-4 = 1.59 × 106 N/m2 b) Area of brake pads = (30 × 4) cm2. Since pressure in the wheel cylinder is the same as in the master cylinder) F = Pressure × Area = (1.59 × 106) × (120 × 10-4) = 1.91 × 104 N. Physics Notes Form 1 in PDFFeatured Physics Form 1 Topics
|
Archives
December 2024
Categories
All
Physics notes form 1 to 4
|
||||||||||||||||||||||||||||||||||||||
We Would Love to Have You Visit Soon! |
Hours24 HR Service
|
Telephone0728 450425
|
|
8-4-4 materialsLevels
Subjects
|
cbc materialsE.C.D.E
Lower Primary
Upper Primary
Lower Secondary
Upper Secondary
|
teacher support
Other Blogs
|






 RSS Feed
RSS Feed